Electric field

$$ E(r) \approx \frac{e^{-jkr}}{r} F(\theta, \phi) $$ Where F is the field vector and it is in Volts.

Radiation power density

The average power radiated by an antenna in a surface comes from the pointing vector (that is a power density ): $$ P_{rad} = P_{av} = \int \int_S W_{av} \cdot \hat n \ da = \frac{1}{2} \int \int_S Re(E \times H^) \cdot ds = [Watts] \\ W_{av} = \text{Time average Poynting vector (average power density)} $$ The pointing vector is defined as: $$ S = \frac{1}{2} E \times H^ = \bar S + jQ $$

$$ W_{av} \approx \hat a_r \frac{1}{2 \eta} \lvert E \lvert^2 = \hat a_r \frac{1}{2 \eta r^2} \lvert F \lvert^2 $$

Radiation intensity

Solid angle is defined as:

$$ d \Omega = \frac{A}{r^2} $$

$$ dP_{rad} = W_{av} \cdot \hat a_r \ dA = W_{av} \cdot \hat a_r r^2 \ d \Omega $$

Radiation intensity in a given direction is defined as “the power radiated from an antenna per unit solid angle.” The normalized radiation intensity is the antenna pattern, which characterizes the antenna. It is usually plotted in linear scale or in dBi.

$$ U = \frac{dP_{rad}}{d \Omega} = W_{av} \cdot \hat a_r r^2 = [W / \text{unit solid angle}] = \frac{1}{2 \eta} \lvert F(\theta, \phi) \lvert^2 $$ The U is the electric field without the R, or the radiation intensity. It is also written like F in some places, that makes it confussing. Where F is the field vector

The radiation intensity of an isotropic source is:

$$ U_0 = \frac{P_{rad}}{4 \pi} $$

Directivity

Directivity (of an antenna) (in a given direction) The ratio of the radiation intensity in a given direction from the antenna to the radiation intensity averaged over all directions. $$ D = \frac{4 \pi U}{P_{rad}} \\ D_{max} = \frac{4 \pi U_{max}}{P_{rad}} $$ In terms of poynting vector, power density, it can be express as:

$$ D = \frac{S_{max}}{S_{av}} = \frac{4 \pi r^2 S_{max}}{\bar P_{rad}} $$ Or in terms of radiation intensity:

$$ D = \frac{F_{max}}{F_{av}} $$

Power in transmitting systems

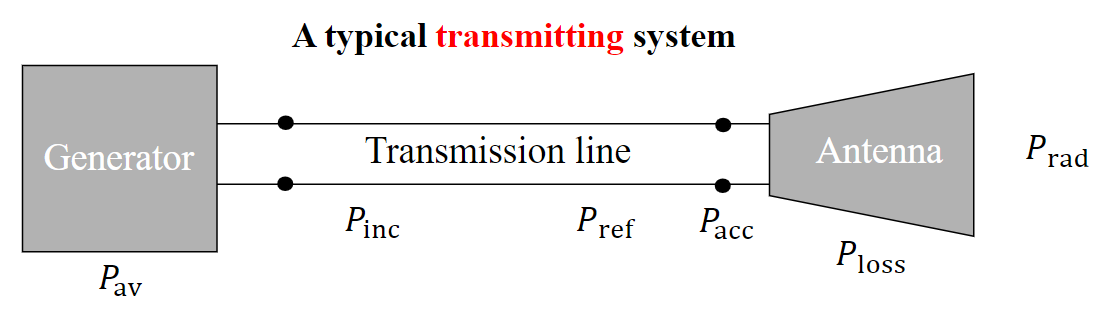
• Available power: Maximum power that the generator can deliver to an external load
• Incident power: Power that the generator would deliver to an infinite transmission line
• Accepted power: Power that the antenna accepts / that is delivered to the antenna
• Reflected power: Difference between incident and accepted powers
• Dissipated power: This is the power that is lost in the antenna and this not radiated
• Radiated power: This is the power that the antenna radiates

$$ P_{acc} = (1 - \lvert \Gamma \lvert^2)P_{inc} \\ P_{ref} = \lvert \Gamma \lvert^2 P_{inc} \\ \Gamma = \frac{V_{reflected}}{V_{fordward}} \\ \Gamma = \frac{Z_L - Z_0}{Z_L + Z_0} \\ VSWR = \frac{1 + \lvert \Gamma \lvert}{1 - \lvert \Gamma \lvert} $$

$$ P_{rad} = \eta_{rad} P_{acc} \\ \eta_{rad} = \text{Radiation efficiency} $$ $$ \eta_A = \frac{R_{rad}}{R_{rad} + R_{loss}} \\ Z_A = \text{Antenna impedance} = R_A + j X_A = R_{rad} + R_{loss} + jX_{A} $$

$$ P_{av} = \frac{\lvert V_g \lvert^2}{8 R_g} $$

Gain of an antenna

Gain of an antenna (in a given direction) is defined as “the ratio of the intensity, in a given direction, to the radiation intensity that would be obtained if the power accepted by the antenna were radiated isotropically.The radiation intensity corresponding to the isotropically radiated power is equal to the power accepted (input) by the antenna divided by \(4 \pi\).

$$ G(\theta, \phi) = 4 \pi \frac{U(\theta, \phi)}{P_{acc}} \qquad G(\theta, \phi) = \eta_{rad} D(\theta, \phi) $$

Polarization

We can define a plane wave travelling through the z axis as:

$$ E(z, t) = \hat a_x E_x(z, t) + \hat a_y E_y(z, t) \\ E_x(z ,t) = Re[E_{x0} e^{j(\omega t + kz + \phi_x)}] $$

Linear polarization

Is linear when:

$$ \Delta \phi = \phi_y - \phi_x = n \cdot \pi $$

Circular polarization

$$ \lvert E_x \lvert = \lvert E_y \lvert \\ \Delta \phi = \phi_y - \phi_x = (\frac{1}{2} + 2 n)\pi $$

Eliptical polarization

$$ \lvert E_x \lvert \neq \lvert E_y \lvert \\ \Delta \phi = \phi_y - \phi_x = (\frac{1}{2} + 2 n)\pi $$

Polarization mismatch

When transmittin in horizontal, or vertical, polarization we can have polarization mismatch when the angles are not the same. This is:

$$ PLF = \lvert \hat \rho_w \cdot \hat \rho_a \lvert^2 = \lvert \cos \phi_p \lvert^2 $$

Where \(\hat \rho_w\) is the unit vector of the receiving wave and (\hat \rho_i\) of the incident wave. The \(\phi_p\) defines the angle between the both polarizations.

Effective area

Is defined as the ratio of the available power at the terminals of a receiving antenna to the power flux density of a plane wave incident on the antenna from that direction, the wave being polarization-matched to the antenna. If the direction is not specified, the direction of maximum radiation intensity is implied.

$$ A_e = \frac{P_{av}}{W_{inc}} = \frac{\lvert V_g \lvert^2}{8 R_A W_{inc}} = [m^2] \\ $$

Where \(W_{inc}\) is the power density of the incident wave, expressed in \([W / m^2]\).

$$ G = 4 \pi \frac{A_e}{\lambda^2} \\ A_e = \eta_{rad} D_r(\theta_r, \phi_r) \Big (\frac{\lambda^2}{4 \pi} \Big ) $$

Friis Trasmission equation

The receiver power densitiy at a given R of an isotropic antennais:

$$ W_0 = \eta_{rad} \frac{P_{acc}}{4 \pi R^2} $$

The power density in a certain direction is:

$$ W_t = \frac{P_{acc} \cdot G_t(\theta_t, \phi_t)}{4 \pi R^2} = \eta_{rad} \frac{P_{acc} \cdot D_t(\theta_t, \phi_t)}{4 \pi R^2} $$

The power received can be expressed as:

$$ P_{av,r} = A_e \cdot W_t = \eta_{pol} P_{acc_t} G_t G_r \Big ( \frac{\lambda}{4 \pi R} \Big )^2 \\ $$